JEE Advanced 2018 Paper 2, Question 4
A wire is bent in the shape of a right angled triangle and is placed in front of a concave mirror of focal length ![]() , as shown in the figure. Which of the figures shown in the four options qualitatively represent(s) the shape of the image of the bent wire? (These figures are not to scale.)
, as shown in the figure. Which of the figures shown in the four options qualitatively represent(s) the shape of the image of the bent wire? (These figures are not to scale.)
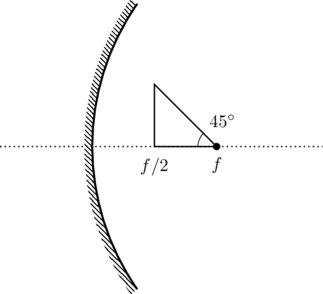
Related article: Optics without ray diagrams: Mirrors
Solution
If the object is kept between a concave mirror and its focal point the image is virtual and upright (see this note). Recall that an object at a distance ![]() from a mirror of focal length
from a mirror of focal length ![]() forms an image at
forms an image at ![]() , such that
, such that
(1) ![]()
The given object is extensive, that is, different points of the object are located at different ![]() . The point
. The point ![]() is at
is at ![]() (see figure), which means there will be a portion of the image at
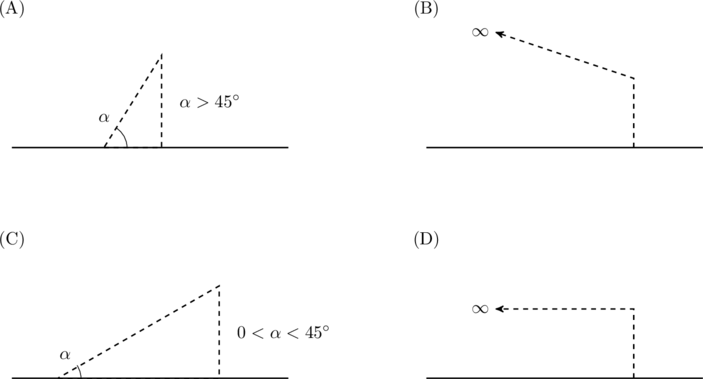
(see figure), which means there will be a portion of the image at ![]() . Therefore, the correct option must be either (B) or (D). To narrow it down further let us consider the segment
. Therefore, the correct option must be either (B) or (D). To narrow it down further let us consider the segment ![]() . The point
. The point ![]() is
is ![]() and
and ![]() is
is ![]() (the triangle is isoceles, which means
(the triangle is isoceles, which means ![]() , but we will continue calling this height
, but we will continue calling this height ![]() for the time being). An arbitrary point on the segment
for the time being). An arbitrary point on the segment ![]() is given by
is given by
(2) ![]()
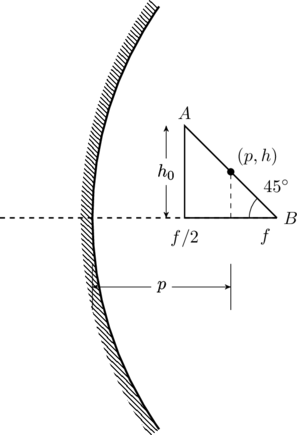
This point will be located at a distance ![]() given by (1),
given by (1),
(3) ![]()
with a height ![]() , where
, where ![]() is the magnification. That is,
is the magnification. That is,
(4) ![]()
which is a constant. Therefore all points on ![]() appear at the same height in the image. Therefore, the correct answer is (D).
appear at the same height in the image. Therefore, the correct answer is (D).
Discussion: The points on the pricipal axis between ![]() and
and ![]() will also be be in the image, although this is not clear from the picture given in the exam. A better illustration would be the figure below.
will also be be in the image, although this is not clear from the picture given in the exam. A better illustration would be the figure below.

Also note that ![]() for any height
for any height ![]() (within the field of view of the mirror, of course). That is, the image has the shape shown above for any
(within the field of view of the mirror, of course). That is, the image has the shape shown above for any ![]() , and not just
, and not just ![]() .
.