Terminal velocity in a magnetic field

A copper connector of mass $m$ slides down two smooth copper bars, set at an angle $\alpha$ to the horizontal, due to gravity (see figure). At the top the bars are interconnected through a resistance $R$ . The separation between the bars is $\ell$ . The system is located in a uniform magnetic field of induction $B$ , perpendicular to the plane in which the connector slides. The resistance of the bars, the connector and the sliding contacts, as well as the self-inductance of the loop are assumed to be negilible. If the connector is released from rest at $t=0$ ,

Find the velocty 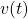$v(t)$ of the connector for all $t>0$ ,
Find the steady-state velocity of the connector,
If the resistance 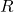$R$ is replaced by a capacitor of capacitance 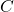$C$ , how does the connector move?
Give a physical explanation of why the motion with 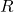$R$ is different from the motion with $C$ ?

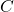(This problem is a slightly modified version of 3.296 and 3.297 of Irodov.)

Solution

There are three forces at work in this problem:

the force of gravity, of which a component 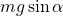$mg \sin \alpha$ pulls the slider down the copper bars,
an electromotive force 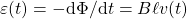$\varepsilon(t) = -{\rm d} \Phi/{\rm d} t = B \ell v(t)$ which drives a current $I(t) = \varepsilon(t) / R$ through the circuit, and
the Lorenz force 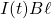$I(t) B \ell$ which tries to keep the connector from sliding down.

The last point warrants further explanation. How do we know that the Lorenz force tries to slow down the slide? It simply follows from Lenz’s law: the circuit will do whatever it can to oppose the change in flux through the loop, which in this case means deter the connector from sliding down. We can combine the above forces to obtain the equation of motion for the connector,

(1) $\begin{align*} m \frac{{\rm d} v}{{\rm d} t} &= m g \sin \alpha - I B \ell \nonumber \\ &= m g \sin \alpha - \frac{B^2 \ell^2 v}{R} . \end{align*}$

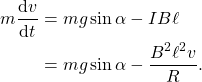
This is a first order differential equation for $v(t)$ with the initial condition $v(0) = 0$ . Notice that a differential equation of the form $\dot f = c f$ is easier to solve ( $c$ is some constant). So we put (1) in that form by defining a new variable $V(t) = -g \sin \alpha + B^2 \ell^2 v/mR$ , which has the derivative

(2) $\begin{equation*} \frac{{\rm d} V}{{\rm d} t} = \frac{B^2 \ell^2}{m R} \frac{{\rm d} v}{{\rm d} t} . \end{equation*}$

Therefore (1) can be written in terms of $V$ as

(3) $\begin{equation*} m \left( \frac{m R}{B^2 \ell^2} \frac{{\rm d} V}{{\rm d} t} \right) = -m V \implies \frac{{\rm d} V}{{\rm d} t} = - \frac{B^2 \ell^2}{m R} V . \end{equation*}$

We can integrate this to obtain

(4) $\begin{equation*} \int_{V(0)}^{V(t)} \frac{{\rm d} V}{V} = - \frac{B^2 \ell^2}{m R} \int_0^t {\rm d} t \implies V(t) = V(0) \exp\left[ -\frac{B^2 \ell^2}{m R} t \right] . \end{equation*}$

Reverting back from $V \to v$ , the velocity of the connector is

(5) $\begin{align*} % -g \sin \alpha + B^2 \ell^2 v/mR &= -g \sin \alpha \exp\left[ -\frac{B^2 \ell^2}{m R} t \right] \\ \boxed{ v(t) = \frac{m g \sin \alpha}{B^2 \ell^2/R} \left(1 - \exp\left[ -\frac{B^2 \ell^2}{m R} t \right] \right) } \end{align*}$

where we have used $V(0) = -g \sin \alpha$ . The steady state velocity of the slide can be obtained by taking the $t \to \infty$ limit of this expression,

(6) $\begin{equation*} v_{\rm steady} = \lim_{t \to \infty} v(t) = \frac{m g \sin \alpha}{B^2 \ell^2/R} . \end{equation*}$

Note that $v_{\rm steady}$ can also be obtained directly without first determining the velocity $v(t)$ for all time $t>0$ . If the connector is sliding down at a constant velocity the net force on it must be zero (Newton’s first law). In other words, (1) becomes

(7) $\begin{equation*} m g \sin \alpha - \frac{B^2 \ell^2}{R} v_{\rm steady} = 0 , \end{equation*}$

which gives us (6) again.

Finally, if $R$ were replaced by capacitance $C$ , we’ll have to modify the expression for the current $I$ in (1) to

(8) $\begin{equation*} I = \frac{{\rm d} Q}{{\rm d} t} = C \frac{{\rm d} \varepsilon}{{\rm d} t} = C B \ell \frac{{\rm d} v}{{\rm d} t} . \end{equation*}$

The equation of motion for the slider is then

(9) $\begin{equation*} m \frac{{\rm d} v}{{\rm d} t} = m g \sin \alpha - C B^2 \ell^2 \frac{{\rm d} v}{{\rm d} t} , \end{equation*}$

which tells us that the connector now slides down with a constant acceleration

(10) $\begin{equation*} \frac{{\rm d} v}{{\rm d} t} = \frac{g \sin \alpha}{1 + \frac{B^2 \ell^2 C}{m}} . \end{equation*}$

Why does the connector velocity relax to a steady value in (5) but keep accelerating in (10)? The difference between the two cases is that the resistor is a dissipative element whereas the capactior is not. A steady velocity is reached with the work done on the system by the external force (gravity) is removed at the same rate by some dissipative element. This is what happens if you drop an object into a fluid; eventually it reaches a terminal velocity when the power added to it by gravity is prompty removed by the dissipative forces of the fluid.

When we replace the resistor with an ideal capacitor there is no more dissipation; some of the work done by gravity keeps adding to the energy of the capacitor but there is always some left to accelerate the connector. If the capacitor took up all energy added by gravity at each instant the connector would stop accelerating, which means no more current flows in the circuit (cf. (8)) and the force of gravity acts unchecked, leading to acceleration again. So there is no way a capacitor can remove power from the ciruit at the rate at which gravity introduces it.

Note: In expressions like (5) the argument of the exponential must be a dimensionless number. That means $\frac{B^2 \ell^2}{m R}$ must have the dimensions of $T^{-1}$ . A quick way to check this is to use (1). Dividing througout by $m$ ,

(11) $\begin{equation*} \frac{{\rm d} v}{{\rm d} t} = g \sin \alpha - \frac{B^2 \ell^2 v}{m R} \end{equation*}$

That means $\frac{B^2 \ell^2 v}{m R}$ must have the dimensions of acceleration, $L T^{-2}$ . But the $v$ in this term has dimension $L T^{-1}$ which confirms that $\left[ \frac{B^2 \ell^2}{m R} \right] = T^{-1}$ .

Leave a Reply Cancel reply