Refracting surface with a coating

JEE Advanced 2014 Paper 1, Question 9

A transparent thin film of uniform thickness and refractive index $n_{1}=1.4$ is coated on the convex spherical surface of radius $R$ at one end of a long solid glass cylinder of refractive index $n_{2}=1.5$ , as shown in the figure. Rays of light parallel to the axis of the cylinder traversing through the film from air to glass get focused at distance $f_{1}$ from the film, while rays of light traversing from glass to air get focused at distance $f_{2}$ from the film. Then

Rendered by QuickLaTeX.com

$\left|f_{1}\right|=3 R$
$\left|f_{1}\right|=2.8 R$
$\left|f_{2}\right|=2 R$
$\left|f_{2}\right|=1.4 R$

Related Problem: Lensing by oil on water

Solution

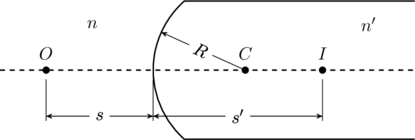
We can solve this problem using the formula for refraction at spherical surfaces (see (27-3) of this lecture or the last equation on this page),

(1) $\begin{equation*} \frac{n}{s} + \frac{n'}{s'} = \frac{n'-n}{R} , \end{equation*}$

In this equation the object is at a distance $s$ from the refracting surface in the medium of index $n$ , and the image is at a distance $s'$ from the same surface in the medium of index $n'$ (see figure below). The sign convention for refracting surfaces are the same as that of lenses.

Rendered by QuickLaTeX.com — A spherical refracting surface.

The present problem can be solved two ways. The first method involves applying (1) twice, once at the surface $A$ separating air from the medium with index $n_1$ , and then at the surface $B$ that separates $n_1$ and $n_2$ (see figure below). This requires no cleverness, but it illustrates how to work with sign conventions for refracting surfaces. So we will try it.

We first consider rays parallel to the axis traveling from air to glass. Such rays are produced by an object kept at infinity. Therefore, at the surface $A$ we have

(2) $\begin{equation*} \frac{1}{\infty} + \frac{n_1}{s_A'} = \frac{n_1 - 1}{R} \implies s_A' = \frac{n_1 R}{n_1-1} . \end{equation*}$

The image at $s_A'$ is the object for the second surface, and is located at $s_B \approx -s_A'$ . This approximation is valid since the medium $n_1$ is thin. The difference in sign between $s_A'$ and $s_B$ is due to the fact that object distances are negative behind the surface whereas image distances are postive there (see this table). Next, we apply (1) on the second surface $B$ ,

(3) $\begin{align*} \frac{n_1}{s_B} + \frac{n_2}{s_B'} = \frac{n_2-n_1}{R} \implies % -\frac{n_1-1}{R} + \frac{n_2}{s_B'} &= \frac{n_2-n_1}{R} \nonumber \\[0.5em] \implies s_B' &= \frac{n_2 R}{n_2-1} \equiv f_1 . \end{align*}$

Next, we keep the object at infinity in the medium $n_2$ . We will take the $n_2$ side as the front of the refracting surface when applying the sign conventions. Refraction through the surface $B$ produces an image at $s_B'$ such that

(4) $\begin{equation*} \frac{n_2}{\infty} + \frac{n_1}{s_B'} = \frac{n_1 - n_2}{-R} \implies s_B' = \frac{n_1 R}{n_2-n_1} \end{equation*}$

Note how the sign of the radius of curvature has changed since the center of the spherical surface is on the object side now (see table). The image at $s_B'$ will be the object for the surface $A$ , at a distance $s_A \approx -s_B'$ . Applying (1) at $A$ ,

(5) $\begin{equation*} \frac{n_1}{s_A} + \frac{1}{s_A'} = \frac{1-n_1}{-R} \implies s_A' = \frac{R}{n_2-1} \equiv f_2 . \end{equation*}$

Substituting $n_2 = 1.5$ in the expressions (3) and (5) we see that $\boxed{f_1 = 3 R, \ f_2 = 2 R}$ . Therefore options (A) and (C) are correct.

Alternate solution: Notice that the expressions (3) and (5) are independent of $n_1$ . This tells us that the coating had no affect on the location of the focal points of the refracting surface $B$ . To understand why, we can consider the coating as a convexo-concave lens (or meniscus) as shown in this figure. However, the radii of curvature of such a lens are equal, $R_1 = R_2 = R$ , which makes the focal length infinity by the Lens Maker’s formula,

(6) $\begin{equation*} \frac{1}{f_A} = (n-1) \left( \frac{1}{R_1} - \frac{1}{R_2} \right) = 0 \implies f = \infty . \end{equation*}$

In other words, the coating has no effect on the overall focal length, since

(7) $\begin{equation*} \frac{1}{f_{\rm eff}} = \frac{1}{f_A} + \frac{1}{f_B} = \frac{1}{f_B} . \end{equation*}$

Therefore, we can calculate $f_1$ and $f_2$ by simply assuming that the coating is absent. Then, the surface $B$ that separates air from $n_2$ , which means parallel rays from air to glass will converge at $f_1$ such that

(8) $\begin{equation*} \frac{1}{\infty} + \frac{n_2}{f_1} = \frac{n_2-1}{R} \implies f_1 = \frac{n_2 R}{n_2 - 1} , \end{equation*}$

and, rays from the glass to air will converge at

(9) $\begin{equation*} \frac{n_2}{\infty} + \frac{1}{f_2} = \frac{1-n_2}{-R} \implies f_1 = \frac{R}{n_2 - 1} . \end{equation*}$

This is exactly the same answer we obtained above, but this approach is quicker.

Leave a Reply Cancel reply