Problem 3.152 of Irodov
The figure below shows an inifite circuit formed by the repetition of the same link, consisting of resistance ![]() and
and ![]() . Find the resistance of this circuit between points
. Find the resistance of this circuit between points ![]() and
and ![]() .
.
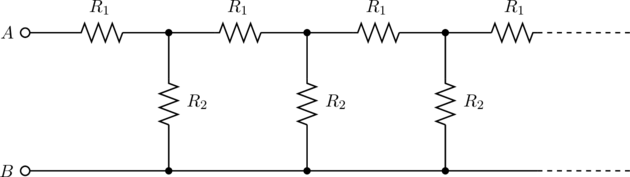
Solution
Let’s denote the resistance between the points ![]() and
and ![]() by
by ![]() . Since the circuit is infinite, removing the first
. Since the circuit is infinite, removing the first ![]() and
and ![]() resistors gives the same arrangement back again — the arrangement is self-similar. That means, the resistance between the points
resistors gives the same arrangement back again — the arrangement is self-similar. That means, the resistance between the points ![]() and
and ![]() is just
is just ![]() without the left-most
without the left-most ![]() and
and ![]() resistors, and we may redraw the circuit as shown below.
resistors, and we may redraw the circuit as shown below.

It is now straightforward to calculate the resistance,
(1) ![]()
which gives a quadratic equation for ![]() ,
,
(2) ![]()
This equation has two roots. We keep only the positive root, because resistance cannot be a negative number.
(3) 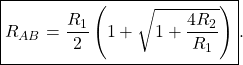
Plugging in ![]() and
and ![]() , we find
, we find ![]() .
.
Bonus Problem: At what value of the resistance ![]() in the circuit shown below will the total resistance between points
in the circuit shown below will the total resistance between points ![]() and
and ![]() be independent of the number of cells. (Hint: What value of
be independent of the number of cells. (Hint: What value of ![]() will make the circuit appear self-similar at the lower rungs of the ladder?)
will make the circuit appear self-similar at the lower rungs of the ladder?)
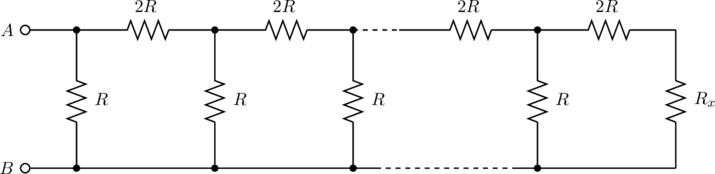
Answer: ![]() .
.